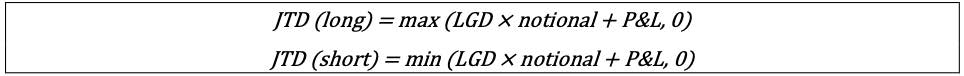Gross jump-to-default risk positions (gross JTD)
| | |
| 8.9 | The gross JTD risk position is computed exposure by exposure. For instance, if a bank has a long position on a bond issued by Apple, and another short position on a bond issued by Apple, it must compute two separate JTD exposures.
| | |
| 8.10 | For the purpose of DRC requirements, the determination of the long/short direction of positions must be on the basis of long or short with respect to whether the credit exposure results in a loss or gain in the case of a default.
| | |
| | (1) | Specifically, a long exposure is defined as a credit exposure that results in a loss in the case of a default.
| |
| | (2) | For derivative contracts, the long/short direction is also determined by whether the contract will result in a loss in the case of a default (ie long or short position is not determined by whether the option or credit default swap (CDS), is bought or sold). Thus, for the purpose of DRC requirements, a sold put option on a bond is a long credit exposure, since a default results in a loss to the seller of the option.
| |
| 8.11 | The gross JTD is a function of the loss given default (LGD), notional amount (or face value) and the cumulative profit and loss (P&L) already realised on the position, where:
| | |
| | (1) | notional is the bond-equivalent notional amount (or face value) of the position; and
| |
| | (2) | P&L is the cumulative mark-to-market loss (or gain) already taken on the exposure. P&L is equal to the market value minus the notional amount, where the market value is the current market value of the position.
| |
|
| 8.12 | For calculating the gross JTD, LGD is set as follows:
| | |
| | (1) | Equity instruments and non-senior debt instruments are assigned an LGD of 100%.
| |
| | (2) | Senior debt instruments are assigned an LGD of 75%.
| |
| | (3) | Covered bonds, as defined within [7.51], are assigned an LGD of 25%.
| |
| | (4) | When the price of the instrument is not linked to the recovery rate of the defaulter (eg a foreign exchange-credit hybrid option where the cash flows are swap of cash flows, long EUR coupons and short USD coupons with a knockout feature that ends cash flows on an event of default of a particular obligor), there should be no multiplication of the notional by the LGD.
| |
| 8.13 | In calculating the JTD as set out in [8.11], the notional amount of an instrument that gives rise to a long (short) exposure is recorded as a positive (negative) value, while the P&L loss (gain) is recorded as a negative (positive) value. If the contractual or legal terms of the derivative allow for the unwinding of the instrument with no exposure to default risk, then the JTD is equal to zero.
| | |
| 8.14 | The notional amount is used to determine the loss of principal at default, and the mark-to-market loss is used to determine the net loss so as to not double-count the mark-to-market loss already recorded in the market value of the position.
| | |
| | (1) | For all instruments, the notional amount is the notional amount of the instrument relative to which the loss of principal is determined. Examples are as follows:
| |
| | | (a) | For a bond, the notional amount is the face value.
|
| | | (b) | For credit derivatives, the notional amount of a CDS contract or a put option on a bond is the notional amount of the derivative contract.
|
| | | (c) | In the case of a call option on a bond, the notional amount to be used in the JTD calculation is zero (since, in the event of default, the call option will not be exercised). In this case, a JTD would extinguish the call option’s value and this loss would be captured through the mark- to-market P&L term in the JTD calculation.
|
| | (2) | Table 1 illustrates examples of the notional amounts and market values for a long credit position with a mark-to-market loss to be used in the JTD calculation, where:
| |
| | | (a) | the bond-equivalent market value is an intermediate step in determining the P&L for derivative instruments;
|
| | | (b) | the mark-to-market value of CDS or an option takes an absolute value; and
|
| | | (c) | the strike amount of the bond option is expressed in terms of the bond price (not the yield).
|
| Examples of components for a long credit position in the JTD calculation | Table 1 | | Instrument | Notional | Bond-equivalent market value | P&L | | Bond | Face value of bond | Market value of bond | Market value - face value | | CDS | Notional of CDS | Notional of CDS -| mark- to-market (MtM) value of CDS | | -| MtM value of CDS | | | Sold put option on a bond | Notional of option | Strike amount -| MtM value of option | | (Strike -| MtM value of option |) - Notional | | Bought call option on a bond | 0 | MtM value of option | MtM value of option | P&L = bond-equivalent market value - notional.
With this representation of the P&L for a sold put option, a lower strike results in a lower JTD loss. |
|
The convertible bonds are not treated the same way as vanilla bonds in computing the DRC requirement Banks should also consider the P&L of the equity optionality embedded within a convertible bond when computing its DRC requirement. A convertible bond can be decomposed into a vanilla bond and a long equity option. Hence, treating the convertible bond as a vanilla bond will potentially underestimate the JTD risk of the instrument.
| | |
| 8.15 | To account for defaults within the one-year capital horizon, the JTD for all exposures of maturity less than one year and their hedges are scaled by a fraction of a year. No scaling is applied to the JTD for exposures of one year or greater.35 For example, the JTD for a position with a six month maturity would be weighted by one-half, while the JTD for a position with a one year maturity would have no scaling applied to the JTD.
| | |
| 8.16 | Cash equity positions (ie stocks) are assigned to a maturity of either more than one year or three months, at banks’ discretion.
| | |
[8.16] states that for the standardised approach DRC requirement, cash equity positions may be attributed a maturity of three months or a maturity of more than one year, at firms’ discretion. Such restrictions do not exist in [13] for the internal models approach, which allows banks discretion to apply a 60-day liquidity horizon for equity sub-portfolios. Furthermore, [8.15] states “... the JTD for all exposures of maturity less than one year and their hedges are scaled by a fraction of a year”. Given the above- mentioned paragraphs, for purposes of the standardised approach DRC requirement, the bank is not permitted to assign cash equities and equity derivatives such as index futures any maturity between three months and one year on a sub-portfolio basis in order to avoid broken hedges As required by [8.16], cash equity positions are assigned a maturity of either more than one year or three months. There is no discretion permitted to assign cash equity positions to any maturity between three months and one year. In determining the offsetting criterion, [8.17] specifies that the maturity of the derivatives contract be considered, not the maturity of the underlying instrument. [8.18] further states that the maturity weighting applied to the JTD for any product with maturity of less than three months is floored at three months. To illustrate how the standardised approach DRC requirement should be calculated with a simple hypothetical portfolio, consider equity index futures with one month to maturity and a negative market value of EUR 10 million (–EUR 10 million, maturity 1M), hedged with the underlying equity positions with a positive market value of EUR 10 million (+EUR 10 million). Both positions in the example should be considered having a three-month maturity. Based on [8.15], which requires maturity scaling, defined as a fraction of the year, of positions and their hedge, the JTD for the above trading portfolio would be calculated as follows: 1/4*10 – 1/4*10 = 0.
| | |
| 8.17 | For derivative exposures, the maturity of the derivative contract is considered in determining the offsetting criterion, not the maturity of the underlying instrument.
| | |
| 8.18 | The maturity weighting applied to the JTD for any sort of product with a maturity of less than three months (such as short term lending) is floored at a weighting factor of one-fourth or, equivalently, three months (that means that the positions having shorter-than-three months remaining maturity would be regarded as having a remaining maturity of three months for the purpose of the DRC requirement).
| | |
In the case where a total return swap (TRS) with a maturity of one month is hedged by the underlying equity, and if there were sufficient legal terms on the TRS such that there is no settlement risk at swap maturity as the swap is terminated based on the executed price of the stock/bond hedge and any unwind of the TRS can be delayed (beyond the swap maturity date) in the event of hedge disruption until the stock/bond can be liquidated. The net JTD for such a position would be zero. If the contractual/legal terms of the derivative allow for the unwinding of both legs of the position at the time of expiry of the first to mature with no exposure to default risk of the underlying credit beyond that point, then the JTD for the maturity-mismatched position is equal to zero.
| | |